The Golden Section in Art, Architecture and Music

This section introduces you to some of the amazing applications and occurrences of the Fibonacci series and the Golden Ratio in architecture, art and in music.

The Golden section in architecture

Even from the time of the Greeks, a rectangle whose sides are in the proportion of two neighbouring Fibonacci numbers has been a seen as a pleasing shape. Such rectangles are approximations of the golden rectangle whose sides are in the ratio of 1:Phi = 1:1.618033 or, equivalently, phi:1=0.618033:1. It appears in many of the proportions of the ancient Greek temple, the Parthenon, in Athens, Greece. (There is a replica of the original building (accurate to one-eigth of an inch!) at Nashville which calls itself "The Athens of South USA".)

The Acropolis, in the centre of Athens, Greece, is an outcrop of rock that dominates this ancient city. It's most famous monument, now largely ruined, is the Parthenon, a temple to the goddess "Athena" built around 430/440BC.

The plan shows that the temple was built on a square-root-of-5 rectangle, that is, it is 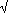sqrt

5 times as long as it is wide. It's front elevation is built on a Golden Ractangle, that is, it is Phi times as wide as it is tall.

Le Corbusier often used golden rectangles in the shapes of the buildings he designed. One of these is the United Nations building in New York which is L-shaped. The upright part of the L has sides in the golden ratio, and there are distinctive marks on this taller part which divide the height by the golden ratio. The United Nations Headquarters On-line Tour has an aerial view of the building (with thanks to Ralph Bechtolt for alerting me to this link).

The Golden Section and Art

Lucas Pacioli (1445-1517) in his Divina proportione (On Divine Proportion) wrote about the golden section also called the golden mean or the divine proportion:

     A     M        B
     | 1-x |    x   |

The line AB is divided at point M so that the ratio of the two parts, the smaller to the larger (AM and MB), is the same as the ratio of the larger part (MB) to the whole AB.

If AB is of length 1 unit, and we let MB have length x, then the definition (in bold) above becomes

the ratio of 1-x to x is the same as the ratio of x to 1 or, in symbols:

     1 - x   =  x  which simplifies to 1-x = x²       x        1

This gives two values for x, (-1-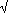 sqrt

5)/2 and ( sqrt

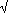5-1)/2.

The first is negative, so does not apply here. The second is just phi (which has the same value as 1/Phi and as Phi-1).

Pacoli's work influenced Leonardo da Vinci (1452-1519) and Albrecht Durer (1451-1578) and is seen in some of the work of Georges Seurat, (for example La Parade) and Paul Signac and Mondrian, for instance.

Many books on oil painting and water colour in your local library will point out that it is better to position objects not in the centre of the picture but to one side or "about one-third" of the way across, and to use lines which divide the picture into thirds. This seems to make the picture design more pleasing to the eye and relies again on the idea of the golden section being "ideal".

Leonardo's Art



The Annunciation: is a picture that looks like it is in a frame of 1:sqrt(5) shape (a root-5 rectangle). Print it and measure it - is it a root-5 rectangle? Divide it into a square on the left and another on the right. (If it is a root-5 rectangle, these lines mark out two golden-section rectangles as the parts remaining after a square has been removed). Also mark in the lines across the picture which are 0.618 of the way up and 0.618 of the way down it. Also mark in the vertical lines which are 0.618 of the way along from both ends. You will see that these lines mark out significant parts of the picture or go through important objects. You can then try marking lines that divide these parts into their golden sections too.


Madonna with Child and Saints: is in a square frame. Print it out and mark on it the golden section lines (0.618 of the way down and up the frame and 0.618 of the way across from the left and from the right) and see if these lines mark out significant parts of the picture. Do other sub-divisions look like further golden sections?

Fibonacci and Poetry

Martin Gardner, in the chapter "Fibonacci and Lucas Numbers" in "Mathematical Circus" (Penguin books, 1979) mentions Prof George Eckel Duckworth's book Structural patterns and proportions in Vergil's Aeneid : a study in mathematical composition (University of Michigan Press, 1962). Duckworth argues that Vergil consciously used Fibonacci numbers to structure his poetry and so did other Roman poets of the time.

Fibonacci and Music

There is a fascinating section in T H Garland's book [see below] on the 5-tone scale (the black notes on the piano), the 8-tone scale (the white notes on the piano) and the 13-notes scale (a complete octave in semitones, with the two notes an octave apart included).

Various composers have used the Fibonacci numbers when composing music - more details in Garland's book.

Golden sections in Violin construction

The section on "The Violin" in The New Oxford Companion to Music, Volume 2, shows how Stradivari was aware of the golden section and used it to place the f-holes in his famous violins.

Baginsky's method of contructing violins is also based on golden sections.

Did Mozart use the Golden mean?

This is the title of an article in the American Scientist of March/April 1996 by Mike Kay. He reports on the analysis of many of Mozart's sonatas and finds they divide into two parts exactly at the golden section point in almost all cases. Was this a conscious choice (his sister said he was always playing with numbers and was fascinated by mathematics) or did he do this intuitively?

Beethoven's FIfth

In an interesting little article in Mathematics Teaching volume 84 in 1978, Derek Haylock writes about The Golden Section in Beethoven's Fifth on pages 56-57.

He finds that the famous opening "motto" appears not only in the first and last bars (bar 601 before the Coda) but also exactly at the golden mean point 0.618 of the way through the symphony (bar 372) and also at the start of the recapitulation which is phi or 0.382 of the way through the piece! He poses the question:

Was this by design or accident?